Computing with only a few light particles

Photons, the particles that makeup light

While it is rarely obvious in day-to-day life, light is made up of discrete particles. This discreteness is what makes light quantum. In fact, the word “quantum” just means that it comes in discrete quantities. As much as it isn’t directly obvious through observation that light comes in discrete particles, this discreteness is important for the physics of the emission of light from hot objects. If light wasn’t composed of discrete particles, the rate of energy emission would be infinite, an apparent paradox known as the ultraviolet catastrophe. This was one of the signs that standard classical physics was incomplete before the development of quantum mechanics. This discretization fixes the apparent paradox, but the subtle mathematical consequences of discretization lead to counterintuitive properties that are the “weirdness” of quantum mechanics. The topic of quantum optics is a fascinating topic that we discuss more fully here. The topic of this discussion is to explain the advantages of computing with only a few light particles, known as photons.

The amount of energy that a photon of a given wavelength (which determines its color) has is fixed, but the amount of energy can be changed by changing the number of photons. A brighter pulse of light contains more photons than a fainter one which is the same color. This gives an obvious motivation to use fewer particles - it requires less energy. One unique aspect that comes from light being quantum is that the number of particles of light can be uncertain. A pulse of light which is represented by a number state contains an exact number of photons. Assuming a perfect measurement device, a number state with two photons will always give a result of exactly two. There is a different kind of state, known as a coherent state, and these states have a fixed amount of energy. This means there will be a fixed amount of photons on average, but measurements will be noisy. Even with a perfect device, if coherent states are measured with an average of two photons, sometimes the observation may only include one photon, sometimes two, sometimes three, sometimes none, etc. The outcomes are random as if each photon has been added independently of the others (mathematically this is known as Poisson statistics). It is therefore only possible to build number states with integer numbers of photons, but coherent states can be made with an average number of photons that are not an integer, and even less than one. A remarkable fact about weak coherent states is that no matter how few photons they have, they still behave like a classical pulse of light until the final measurement, which must measure an integer number of photons. Weak coherent states are relatively easy to produce, so as we see later, they provide a good starting point for developing technology.

It is easier to see quantum mechanics with fewer particles

Since coherent states always behave in a fully classical way, it is natural to ask how we even define a measure of quantum behavior. There are many ways to answer this question, but most follow the general strategy of looking for a case where the classical and quantum models behave differently. For example, some statistical quantities are allowed to take different values quantum mechanically than classically. One example that is relevant to light is something known as a correlation function, which measures how far apart or close together photons are detected on average. Intuitively we can imagine that if photon numbers are measured randomly and proportional to the brightness of the light, then there is no way to make the measurements more regularly spaced out than having a beam of constant brightness. This is because adding, “lumps” or “dips” to the brightness will only mean that on average the detections are closer. For a perfect detector, detecting a photon can only ever make it more likely that we detect another one soon after, not less. This can be seen explicitly in the animation below, no matter what the intensity profile looks like, the probability peaks at a time difference of zero and the most spread out they can be is if the intensity is uniform. The bumps, dips, or even periodic structures just make the distribution more bunched.

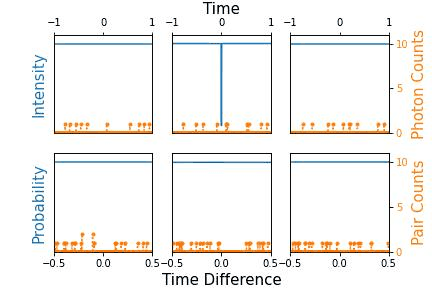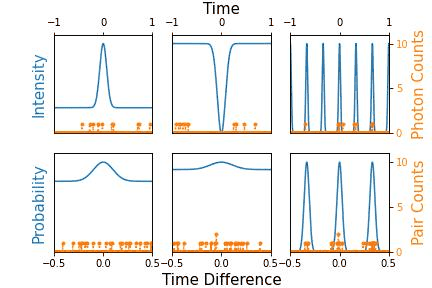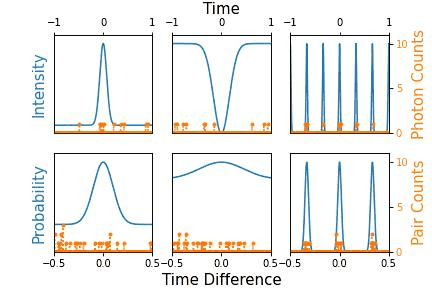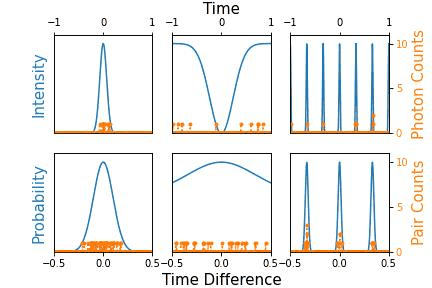
However, if we imagine light as consisting of individual particles, not just a probability distribution for independent measurements, then it is perfectly possible to send the particles one at a time in a spaced-out manner. There is a kind of light known as antibunched light that is less likely to detect a photon right after detecting another one. This early experiment was able to observe antibunching in light from a quantum source. It should be intuitive that evenly spaced single light particles are the way to make light very antibunched indicating the quantum nature of single photon states. A related way to think about the quantum nature of light is by calculating a quantity known as “second-order coherence”. This states that as the number of photons in a number state increases, this quantity approaches, but never quite reaches, the minimum value that classical light can take. Adding more particles, even in a very quantum way, masks the fact that they are discrete particles. This intuitively makes sense, it is hard to tell that a beach is made of millions of grains of sand from a picture. However, if you sprinkle a few grains of sand on the floor it is easy to tell they are discrete separate objects. This is even more obvious for a single grain of sand. Quantum states (as opposed to coherent states, and including but not limited to number states) with a single or few photons are an excellent environment to realize quantum effects, but weak coherent states also have their place. 

Weak coherent states as a testbed and starting point

An advantage of relatively easy-to-prepare weak coherent states is that they provide an excellent tool to understand what we can do and to contribute to the development of our devices, ensuring scalability. While they don’t have the desirable quantum properties, weak coherent states will behave like single photons in many other ways. For example, they are very low energy and are therefore hard to detect and implement non-linear interactions. We can therefore use these states to understand what works and what doesn’t at an earlier stage in hardware development. Additionally, nonlinear optics can also convert weak coherent states into more quantum states. As we discuss elsewhere, nonlinear optics is the key to quantum optics, so a nonlinear system that starts with weak coherent states can transform them into more interesting quantum states over time. 

The nonlinear effects that provide the foundation of our technology have been experimentally demonstrated by our team to make an excellent source of single photons. However, working with weak coherent states allows for a setting where engineering is much easier, and allows us to rapidly test what works and what doesn’t. This ability to “look ahead” allows us to design different elements of our architecture independently, and gain an understanding of encodings and protocols on a large scale early on.